91. Electric field inside the conductor with current

How does an electric field appear in a conductor?

How does an electrical field appear inside a conductor, such as inside a Chicago - Boston cable line?

The necessary condition for an electrical current to appear in a conductor is the existence of voltage at the ends of the conductor. If there is voltage, there is an electric field. But an electric field is created by electric charges. Therefore, charges of the opposite sign must accumulate on the battery terminals or in the electrical socket of your apartment. This means, in turn, that there is some mechanism inside the battery or in the generator of the power plant that drives the charges. Electric current in the circuit is ultimately generated by this mechanism.

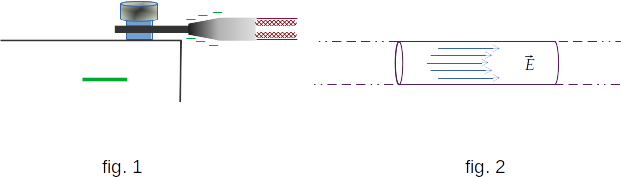
At the first moment after the circuit is closed, the electric field appears only at the ends of the conductor. It causes the electrons in the conductor to move in all directions. The displacement of electrons along the conductor represents the generation of electric current. The movement of electrons across the conductor will continue until they reach the surface of the conductor and form surface charges on it (fig. 1). Surface charges create a field of sufficiently large magnitude in the next section of the conductor. There the process will be repeated: the charges will shift along the conductor, the surface charges will form and therefore the electric field in the next section of the conductor. This process will take place until the surface charge appears along the entire conductor. This charge will create a uniform electric field inside the conductor, directed along the axis (fig. 2). (It can be proved that the field will indeed be homogeneous for not too high currents when the magnetic interaction can be neglected). There can be no field in the opposite direction, since in this direction the charges only move at the first moment when the field is set in the conductor.

The velocity of the ordered motion of electrons.

The process of establishing the electric field moves along the conductor at a speed close to the speed of light, \(300\,000 \,km/sec\). The electrons themselves move along the conductor very slowly. The velocity of their movement is easy to find. According to \((9-3)\) this velocity is equal to

\( \upsilon \,= \frac{j}{q\,n} \)

Let, for example, the density of electric current \(j \,= \,10 \,\frac{k}{m^2\,sek}\) (it corresponds to the current of \(1 amperes\) in a conductor with the cross-section of \(1 \,mm^2\)). The charge of the electron by value \(\,q \,= \,e \,= \,1.6 \cdot 10^{-19}k\).

Number of free electrons in a cubic centimeter of a conductor (for example, copper) we will find as follows.

When a piece of copper is formed, one of the valence electrons of each copper atom collects and becomes free.
The number \(n\) is the number of atoms in \(1\,m^3\) of copper.
A kilogram atom of copper \(\mu \,= \,63.5 \,kg/kmol.\)
Its density is \(\rho \,\approx \,9000 \,kg/m^3\).
The volume of the kilogram atom \(V_{\mu} \,= \,\frac{\mu}{\rho}.\)
As number Avogadro \(N_A \,= \,6 \cdot 10^{26} \,kmol^{-1}\), then

\( n \,= \,\frac{N_A}{V_\mu} \,= \,\frac{N_A}{\mu} \,\rho \)

Hence, \(\,\upsilon \,= \,\frac{j\,\mu}{e\,N_A\,\rho} \,= \, 7\cdot 10^{-5} \frac{m}{sek} \,= \,0.07\frac{mm}{sek}.\)

We see that the average velocity of directed motion of electrons is very small. It increases with increasing density of electric current.