89. Electric current branching

Very often in electric circuits several (more than two) conductors converge at one point. For example, when a voltmeter is included in a circuit (fig. 1), three conductors converge at points \(1\) and \(2\).

Such points are called branching points or nodes.

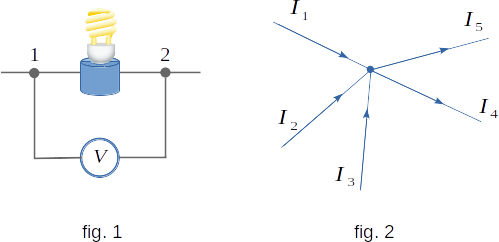
In figure 2, the five conductors converge at the branching point. Let us denote the current forces in conductors \(I_1, \,I_2, \,I_3, \,I_4 \,and \,I_5\).

If direct currents flow through the conductors, no electrical charge can accumulate in the node because the accumulation of charge would change the electrical field and therefore the force acting on the moving charges. In this case, the forces of currents would change. Therefore, the quantity of charge delivered to the node with currents \(I_1, \,I_2, \,I_3\,\) in the one unit of time entering to the node, should be equal to the charge carried for the same time by currents \(I_4, \,I_5\,\) from the node. Consequently, it is fair to equal

\( I_1 \,+ \,I_2 \,+ \,I_3 \,= \,I_4 \,+ \,I_5 \)

or

\( I_1 \,+ \,I_2 \,+ \,I_3 \,- \,I_4 \,- \,I_5 \,= \,0 \)

Considering the current force as an algebraic value having the "plus" sign if the current enters the branching point, and the "minus" sign if it leaves the branching point, the last equation can be written so

\( \sum\limits_{i=1}^{5} I_i \,= \,0 \)

If \(N\) currents converge in the node, then

\( \sum\limits_{i=1}^{N} I_i \,= \,0 \)

The algebraic sum of electric current forces in conductors converging at the branching point is equal to zero.